limit comparison test hard questions|9.4: Comparison Tests : wholesaling 10.6 Integral Test; 10.7 Comparison Test/Limit Comparison Test; 10.8 . WEBTodo sobre el equipo Atl. Chiriquí (Liga Panameña Clausura) plantilla actual con valores de mercado fichajes rumores estadísticas jugadores Calendario de juego noticias Atlético Chiriquí - Perfil del club | Transfermarkt
{plog:ftitle_list}
web11 de fev. de 2023 · Escalações prováveis. Água Santa – Técnico: Thiago Carpini. Lucas Tocantins, fora do último jogo com um desconforto muscular, deve ser opção no banco de reservas. Provável escalação: Ygor Vinhas; .
Here is a set of practice problems to accompany the Comparison Test/Limit Comparison Test section of the Series & Sequences chapter of the notes for Paul Dawkins Calculus II course at Lamar University.

10.6 Integral Test; 10.7 Comparison Test/Limit Comparison Test; 10.8 .Here is a set of assignement problems (for use by instructors) to accompany the .
In this section we will discuss using the Comparison Test and Limit Comparison .
In this section we will discuss using the Comparison Test and Limit Comparison Tests to determine if an infinite series converges or diverges. In order to use either test the .For others, simple comparison doesn’t work quite right and instead you must use the Limit Comparison Test. For each of the following, determine what known series to compare to, and .Direct Comparison Test for Series: If 0 ≤ a n ≤ b n for all n ≥ N, for some N, then, 1. If X∞ n=1 b n converges, then so does X∞ n=1 a n. 2. If X∞ n=1 a n diverges, then so does X∞ n=1 b n. The .
Use the Limit Comparison Test to determine whether each series in exercises 14 - 28 converges or diverges. 14) \(\displaystyle \sum^∞_{n=1}\left(\frac{\ln n}{n}\right)^2\) AnswerThen use the comparison test or the limit comparison test (in some cases, only one of these will work; in other cases, either method will work) to determine whether each series converges .How to use the limit comparison test to determine whether or not a given series converges or diverges, examples and step by step solutions, A series of free online calculus lectures in videos
Learning Outcomes. Use the limit comparison test to determine convergence of a series. The comparison test works nicely if we can find a comparable series satisfying the hypothesis of .The limit comparison test. We compare infinite series to each other using limits. Using the comparison test can be hard, because finding the right sequence of inequalities is difficult. The . Use the comparison test to test a series for convergence. Use the limit comparison test to determine convergence of a series. We have seen that the integral test .Use the Limit Comparison Test to determine whether each series in exercises 14 - 28 converges or diverges. 14) \(\displaystyle \sum^∞_{n=1}\left(\frac{\ln n}{n}\right)^2\) . Argue that this depleted harmonic series converges by answering the following questions. a. How many whole numbers \(n\) have \(d\) digits? b. How many \(d\)-digit .
Limit Comparison Test. The comparison test works nicely if we can find a comparable series satisfying the hypothesis of the test. However, sometimes finding an appropriate series can be difficult. Consider the series \[\sum_{n=2}^∞\dfrac{1}{n^2−1}.\] It is natural to compare this series with the convergent series \[\sum_{n=2}^∞\dfrac{1}{n . Here is a set of assignement problems (for use by instructors) to accompany the Comparison Test/Limit Comparison Test section of the Series & Sequences chapter of the notes for Paul Dawkins Calculus II course at Lamar University.
Worksheet 15
This theorem should make intuitive sense. If then we have for large , so the behavior of the respective series should be the same.; If then should be way less than .So if converges, should also converge by the comparison test.; If , then should be way greater than .So if diverges, should also diverge by the comparison test.; The way we actually use this in practice still . The regular Comparison Test for Series was good, but it had a crucial problem: often we can't actually find an easy inequality despite being able to eyeball . This test is pretty straightforward. In our notation, we say that the series that you are trying to determine whether it converges or diverges is and the test series that you know whether it converges or diverges is .The limit has to be calculated for you come to any conclusion. Also, notice that the fraction can be inverted and the test still works for case 1 (but .Math: Pre-K - 8th grade; Pre-K through grade 2 (Khan Kids) Early math review; 2nd grade; 3rd grade; 4th grade; 5th grade; 6th grade; 7th grade; 8th grade; See Pre-K - 8th Math
Melting Point Tester fabrication
Example 2 Use the comparison test to determine if the following series converges or diverges: X1 n=1 21=n n I First we check that a n >0 { true since 2 1=n n >0 for n 1. I We have 21=n = n p 2 >1 for n 1. I Therefore 2 1=n n >1 n for n >1. I Since P 1 n=1 1 is a p-series with p = 1 (a.k.a. the harmonic series), it diverges. I Annette Pilkington .The limit comparison test is a good substitute for the comparison test when the inequalities are difficult to establish; essentially, if you have a feel that the series in front of you is "essentially proportional" to another series whose convergence you know, then you can try to use the limit comparison test for it.
Solution: Limit Comparison Test. Let a n = 1 en and b n = 1 en n2 (both positive). lim n!1 a n b n = lim n!1 en n2 en = 1 lim n!1 n2 en = 1 (By L’Hopital’s Rule). The limit is nite and not zero, so the Limit Comparison Test applies. X1 n=1 1 en = X1 n=1 1 e n is a convergent geometric series (r = 1 e < 1). Therefore, by the Limit Comparison .
Laboratory Turbidimeter fabrication
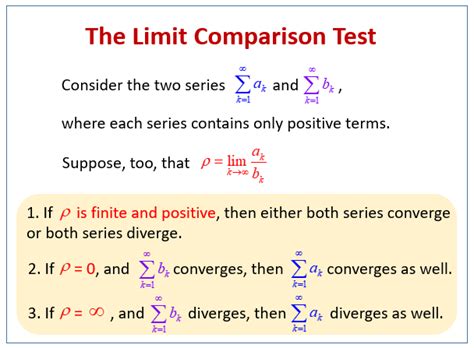
The limit comparison test
10.6 Integral Test; 10.7 Comparison Test/Limit Comparison Test; 10.8 Alternating Series Test; 10.9 Absolute Convergence; 10.10 Ratio Test; 10.11 Root Test; 10.12 Strategy for Series; 10.13 Estimating the Value of a Series; 10.14 Power Series; 10.15 Power Series and Functions; 10.16 Taylor Series; 10.17 Applications of Series; 10.18 Binomial . The Ratio Test takes a bit more effort to prove. 5 When the ratio \(R\) in the Ratio Test is larger than 1 then that means the terms in the series do not approach 0, and thus the series diverges by the n-th Term Test. When \(R=1\) the test fails, meaning it is inconclusive—another test would need to be used.Using the comparison test can be hard, because finding the right sequence of inequalities is difficult. . The limit comparison test does not apply because the limit in question does not exist. The comparison test can be used to show that the original series converges.
Integral Test; Direct Comparison Test; Large Limit Comparison Test; Contributors and Attributions; Knowing whether or not a series converges is very important, especially when we discusses Power Series. Theorems 60 and 61 give criteria for when Geometric and \(p\)-series converge, and Theorem 63 gives a quick test to determine if a .
It is hard to apply the Limit Comparison Test to series containing factorials, though, as we have not learned how to apply L’Hôpital’s Rule to n!. Example 9.4.5 Applying the Limit Comparison Test. 10.6 Integral Test; 10.7 Comparison Test/Limit Comparison Test; 10.8 Alternating Series Test; 10.9 Absolute Convergence; 10.10 Ratio Test; 10.11 Root Test; 10.12 Strategy for Series; 10.13 Estimating the Value of a Series; 10.14 Power Series; 10.15 Power Series and Functions; 10.16 Taylor Series; 10.17 Applications of Series; 10.18 Binomial .Question: Explain how the Limit Comparison Test works. Choose the correct answer below. O A. Find an appropriate comparison series. Then determine whether the terms of the given series are less than or equal to or greater than or equal to for all large values of k. This comparison determines whether the series converges. OB.
The Limit Comparison Test Proof of the Limit Comparison Test: First we assume that lim n!1 a n b n = L 1) If 0 < L < 1, the interval (L 2;2L) is an open interval containing L. It follows that we can find a cutoff N2N so that if n , then L 2 < a n b n < 2L or equivalently that L 2 b n < a n < 2Lb n If P1 n=1 a n converges, then the Comparison .
10.6 Integral Test; 10.7 Comparison Test/Limit Comparison Test; 10.8 Alternating Series Test; 10.9 Absolute Convergence; 10.10 Ratio Test; 10.11 Root Test; 10.12 Strategy for Series; 10.13 Estimating the Value of a Series; 10.14 Power Series; 10.15 Power Series and Functions; 10.16 Taylor Series; 10.17 Applications of Series; 10.18 Binomial . 10.6 Integral Test; 10.7 Comparison Test/Limit Comparison Test; 10.8 Alternating Series Test; 10.9 Absolute Convergence; 10.10 Ratio Test; 10.11 Root Test; 10.12 Strategy for Series; 10.13 Estimating the Value of a Series; 10.14 Power Series; 10.15 Power Series and Functions; 10.16 Taylor Series; 10.17 Applications of Series; 10.18 Binomial .
10.6 Integral Test; 10.7 Comparison Test/Limit Comparison Test; 10.8 Alternating Series Test; 10.9 Absolute Convergence; 10.10 Ratio Test; 10.11 Root Test; 10.12 Strategy for Series; 10.13 Estimating the Value of a Series; 10.14 Power Series; 10.15 Power Series and Functions; 10.16 Taylor Series; 10.17 Applications of Series; 10.18 Binomial . Well, I would try to see if I can directly compare first; however, it might not be easy when its expression is complicated. The benefit of the limit comparison test is that we can compare series without verifying the inequality we need in order to apply the direct comparison test, of course, at the cost of having to evaluate the limit. In this video, we are dealing with a series with rational terms. It's hard to compare using direct comparison. So, we are going to using the Limit Comparison. Section 10.7 : Comparison Test/Limit Comparison Test. Back to Problem List. 7. Determine if the following series converges or diverges. \[\sum\limits_{n = 0}^\infty {\frac{{{2^n}{{\sin }^2}\left( {5n} \right)}}{{{4^n} + {{\cos }^2}\left( n \right)}}} \] . we have in this series that they are positive and so we know that we can attempt the .
The Limit Comparison Test (examples, solutions, videos)
For problems 11 { 22, apply the Comparison Test, Limit Comparison Test, Ratio Test, or Root Test to determine if the series converges. State which test you are using, and if you use a comparison test, state to which other series you are comparing to. 11. X1 k=1001 1 3 p k 10 The series diverges by the Comparison Test. Compared to X1 k=1001 1 3 .In mathematics, the limit comparison test (LCT) (in contrast with the related direct comparison test) is a method of testing for the convergence of an infinite series. Statement. Suppose that we have two series .
Tour Start here for a quick overview of the site Help Center Detailed answers to any questions you might have Meta Discuss the workings and policies of this site
.jpg)
Resultado da Slots of Vegas Casino No Deposit Bonuses 2024 EXCLUSIVE $25 No Deposit Bonus Code $2500 deposit bonus + 50 Free Spins
limit comparison test hard questions|9.4: Comparison Tests